There is a myth built around the so-called House Edge at online and live roulette. It is time to dispel it once and for all. This is going to be the definitive text about the house edge in roulette. A monograph that clearly and fully explains what is the house edge, the theoretical arguments build on it and the reality of how it actually affects your play and winnings at the roulette tables.

Why is it so difficult to win at roulette? If you pose this question to a mathematician or post it into a gamblers forum, the usual answer you will receive is that roulette is not just difficult, but impossible to beat in the long run. Now, no one would doubt that you can win at roulette in a couple of visits to the casino. But they are sure that you cannot stay in profit for too long. “Hey! I have a nice bet selection and great progression, so maybe I can be profitable” you might say. “No chance!” they would answer. Either you use a refined system or throw your chips at random numbers, the outcome, according to those guys, will be the same: Loss. Not only do they know that you will lose in the long run, they also know how much you will lose: 2,7% of your bets. Yep! No matter how you play “in the long run you will be in loss for 2,7% of the total amount of money you ever bet on roulette! If you ask them how do they know the outcome beforehand and why are they so sure of it, they will answer monotonously: “It is the House Advantage stupid!” But they are wrong!

The main idea behind the house advantage (or house edge or player dis-advantage or average expectation – they all refer to the same thing) is that the casinos need to make money. Therefore every casino game has a specific house advantage, depending on the type of the game (roulette, blackjack, craps, Texas Holdem etc.). Theoretically, the house advantage is the amount of money the casino is making for itself, while the rest of the money is given back to the players by paying out their wins. Another way to describe the house edge is that from all the bets that take place on a roulette table, the casino will pay-out to the players all the money played minus a small percentage, that percentage is called House Edge.

Let’s be more specific. As you know roulette has, on the table layout and on the actual roulette wheel, the 37 numbers from 0 to 36.[*] Of these 37 numbers the 36 (1-36) are colored black or red and the Zero is colored green. The Green zero symbolizes the house edge. And I say “it symbolizes” and not “it is” the house edge, because if we want we can freely bet on zero and get a profit from it. But the point is that although the roulette wheel and table have 37 numbers and 37 discrete possible outcomes, we are paid as if it had only 36. This means that our payout in case of win is 1/37 = 2,7% lower than it should.

When you bet on RED even chance, it is actually not exactly an even chance, since on the wheel there are 18 red numbers, 18 black numbers and 1 zero. So you have 18/37 = 48,7% chance of winning (and not 50%), therefore your payout should have been (37-18)/18= 19/18= 102,7% of your bet. But you are paid only 100% of your bet.

When you bet a single number, your odds of winning at roulette is 1/37 = 2,7% and your payout should have been (37-1)/1=36= 3600% or 36 times your bet. But you are paid only 3500% or 35 times your bet. This is a 2,7% difference of the ideal payout.

There is no doubt: although the roulette wheel has 37 numbers, casinos pay us as if roulette only had 36 numbers and this means they pay us 2,7% less than it is “fair”. This “unfair” 2,7% goes to the casino, to pay their bills and this is the so called the House Edge or House Advantage.

The mathematicians take the concept of the house edge to a whole new level and they conclude that it is impossible to win at roulette in the long run. Their reasoning is as follows:

No matter what is your roulette strategy, the house advantage cannot be negated. It is always there no matter what combination of bets you choose. Let’s say for example that you bet $5 on zero (0) and $20 on two dozens. This is 68% probability of winning. But let’s calculate the risk/reward relation. You have 1/37 chance of winning $135 (for the zero), 24/37 chance of winning $15 (for the 2 dozens), and 12/37 chance of losing $45 (for the third dozen). The overall expected return or expected value is:

So they prove that the player disadvantage is there, steady at -2,7% on every bet. And therefore they arbitrarily conclude that roulette strategies or progressions or bet selections make no difference and offer no advantage.

Then they go a bit further and they say, arbitrarily again, that since every bet on the roulette table has a negative average value (-2,7%), then in the end (in the long run), one will inevitably lose an amount of money, on the average, equal to 2,7% of all his life bets. They call that average expectation.

Their verdict: You can not win at roulette, because it is a negative expectation game.
Now let’s see why they are wrong. Why this is too much narrow-minded mathematics and too little real life.

I want to emphasize that the house advantage is a considerable disadvantage for any roulette player. It eats up part of our winnings. And yes, if it didn’t exist, our lives as gamblers would be much easier. But generally speaking, its significance is greatly overstated. It is not the “be all end all” ultimate player killer and its existence constitutes no prof that one can not be profitable with the ideal strategy. Here is why:

• There is not and there can never be any mathematical proof that a roulette strategy cannot be profitable. This is impossible because roulette is a random game and none can guarantee (let alone prove) that you will lose.

• Υou can think of the house edge as a minimal 2,7% tax, automatically applied on your wins, every time you win. To make some analogies to the real world, the math boys argument is like saying that you cannot be profitable in business because you have to pay taxes. Indeed, taxes decrease your profit, but cannot stop you from being profitable. It is also like saying that you can’t make money in the stock market because you have to pay a commission for every transaction. Do you understand the nonsense of this way of thinking?

• The “mathematical” approach, ignores blatantly any strategy applied by the player. Either you throw the chips randomly on the table or you play with discipline a very well thought out roulette system, it is considered absolutely the same thing, by the house advantage advocates. Progressions, stop loss, personal permanence, trigger events, bet selection… all these parameters leave them cold. If you play roulette you play roulette; how you play it makes no difference. This is simply not true. The truth is that, because it is not easy to mathematically evaluate the merit of a roulette strategy and put a specific numerical value to it, they simply choose to ignore this parameter altogether.

• What also strikes me as amazing is the blind belief in probability expectations when we all know that reality can vary greatly from the theoretically expected results. Being sure that you can not win at roulette just because there is a 2,7% house advantage (player dis-advantage) is like believing that in 111 roulette spins there will be exactly 54 Reds, 54 Blacks and 3 Zeros (Greens), just because that is the theoretical expectation. This not only wrong, it is also a contradiction from the “math guys”. They often tell us that every spin is independent, and “nothing is due” and extreme variations from the average even after thousands of spins are perfectly normal. Yet they religiously believe in the 2,7% disadvantage making us lose like probabilistic zealots.

• I have never met an amateur ot a pro gambler who has lost in his gambling life an amount equal to the house advantage (2,7% of his total bets). Most of them have lost much more and some have lost much less. And I have stopped to care so much about the result of testing millions of spins because I don’t plan to play a million of spins in my life.

• If you told me that today there will be 95 Reds, 100 Blacks and 6 Greens, I could bet on Red with the proper money management and finish the session with profit. And the odds would be against me more than 2,7%. That scenario is not a big problem.

Then why do we lose?

If the negative expectation is not such a big issue, then why do we lose at roulette? A valid question. Indeed, the extreme difficulty to be profitable in roulette, is one of the reasons why the house edge myth is so popular. It is the easy, and “scientific” explanation of why we lose. However it is not the true and complete explanation. Yes, the house advantage doesn’t make things easier, but it can be overcome with a nice progression.

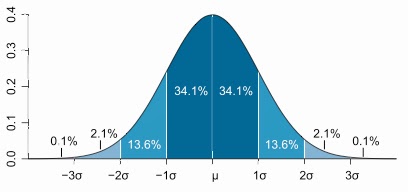
The main reason for the losses of most serious and thoughtful roulette players is something totally different: It is the variance from the average, the difference between theoretical expectation and actual outcomes, the extreme fluctuations. That is the reason we lose. We lose because sometimes we experience crazy spin sequences that fall in the tails of the normal distribution graph. You just cannot beat those roulette spins from hell. It is often said that even the best roulette strategy cannot negate the -2,7% average expectation. But the point of a good system is to overcome a 10 or 15% disadvantage due to variance. Compared to that, the -2,7% is peanuts.

Variance from the average
If there was no zero and no house edge, if you bet on Black, in 100 spins you could theoretically expect 50 Blacks +50 Reds). You would have 50% wins. With the introduction of zero you would expect roughly 48 Blacks (+49 Reds +3Greens). You would have 48% wins. Less than 50% but No big deal! There are many progressions that can produce profit with 48% win rate. The real problem, and the reason we could always lose at roulette is that, no matter what the theoretical expectation, it IS possible to have a 100 spin sequence with only 37 Blacks(!) which is a mere 37% win rate! Now that’s difficult to beat. And such a low hit rate would be possible even if there was no zero and no house edge.

Conclusion

The house edge in roulette is a reality. The roulette player, every time he wins, is being paid 2,7% less than he should. However this is by no means proof that he can not be profitable with the right strategy. And anyway, the house advantage is a minor issue compared with much more important issues the roulette player has to deal with, like the variance from the expected average of spin outcomes.

American roulette

Except from the single zero European roulette there is also the double zero American roulette, which has two zeros (0 and 00) and 38 numbers in total. The payout is exactly the same. The house advantage on the double-zero roulette is 2/38= 5,3%. For simplicity purposes, the above monograph is referring only to the single zero roulette wheel. The logic of the arguments however hold true even for the American wheel. The 5,3% disadvantage of the player is a considerable handicap, but it is still not enough reason to totally dismiss the possibility of winning at roulette with the appropriate strategy.