HOUSE EDGE

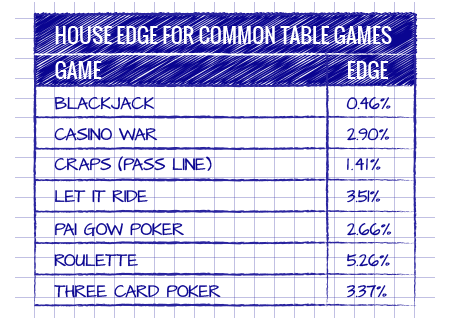
Figure 1 indicates the house edge (or “house advantage,” or “H/A”) for some well known games. The house edge is commonly understood as the percentage of total initial player wagers the casino expects to earn over the long run if all players use perfect strategy against the game. For those games that have exactly one wagering opportunity, the house edge refers to the edge for that wager.

This is the case with the percents given for Casino War, and roulette, as well as keno, slots and video poker games. If a table game has a main game and a side bet, then it is usually the house edge of the main game that is quoted as the house edge for the overall game. For example, Three Card Poker has both the Ante/Play and the Pair Plus wagers; the stated house edge is for the main game, the Ante/Play bet. Blackjack and craps also have more than one wagering opportunity 

Blackjack actually has a second wagering opportunity that very few players use and it is not considered on the house edge. Players, dealers and casino management don’t think about insurance properly; insurance is a separate wagering opportunity in blackjack that is independent of the main bet. It is a side bet. It is a wager that is available to the player any time the dealer shows an Ace. The player is paid 2-to- 1 on the wager if the dealer has a face card under the Ace, otherwise the player loses. There is no theoretical link between the main bet and the insurance bet. The house edge for the insurance wager on an 8 deck game is 7.47%.

Note that even if a strategy is trivial, there will still be player errors. In Three Card Poker, the correct strategy is to raise with Q-6-4 off-suit or higher and to fold all other hands. The strategies for blackjack, Let it Ride and Pai Gow Poker are complex. The more complicated the strategy, the more errors the players will make. Some errors are very costly on simple games. Error rates translate to returns to the casino higher than the “theoretical win” predicted by the house edge.

Pay tables and other features of a slot are created by a mathematician who analyzed the game and built a spreadsheet for the slot machine, called a PARS sheet (PARS = “Pay Table and Reel Strip). A PARS shows the game manufacture how to create the machine by indicating exactly how each reel strip is set up and how much each combination pays when it is hit. A common misconception is that somehow slots are set up to be hot or cold at certain times. In fact, each location on each reel is equally likely to occur on each spin, and this doesn’t change. The changes are set in advance by engineering the design of the reels and the payouts for combinations.

Typically a casino can choose from a menu of possibilities and get a house edge for a slot anywhere from 2% to 20%. Slots that have a house edge under 4% are called “loose.” Slots that have a house edge of 8% or higher are often referred to as “tight.” For video poker games, the game is dealt with a single deck of cards. By law, the deal of these cards must behave statistically in every way just like the game as dealt live. There can be no weighting of the cards or other deviations. Each card must be equally likely to be dealt any time it remains on the deck. Video poker comes in a wide variety of versions. The most common and most popular variants are Jacks or Better, Bonus, Double Bonus, Deuces Wild, and Joker Poker. In each case the house edge is determined by the payouts for various poker hands.

For example, playing Jacks or Better, there is a payout for any final poker hand that consists of a pair of Jacks or higher. Figure 2 gives the pay table that knowledgeable video poker players seek out. The player must play 5 credits to get the listed payouts. Note that these payouts are “for-1.” The player’s credits are deducted from his total before the hand begins. For those who wager less than “max coin” the player receives a lower payout for a Royal Flush, typically 250 instead of 800.

The house edge for this pay table is 0.46%, based on the player playing perfect strategy. A perfect strategy is quite complicated and all but a few experts play a considerably worse game. In practice, a house edge of about 1% is earned by the casino. This pay table is referred to as Jacks or Better “9/6,” with the 9/6 referring to the payouts for a Full House (9) and Flush (6). The most common pay table for Jacks or Better is 8/5, which has a house edge of 2.70% playing perfect jacks or better strategy. At some casinos you will see a 7/5 (house edge = 3.85%) or even a 6/5 (house edge = 5.00%) pay table for Jacks or Better. I have never seen a pay table tighter than 6/5. 

For most video poker games, pay tables exist that have close to a 0% break even house edge, or in some cases, have a slight player edge. For example, 10/6 Jacks or Better (player edge = 0.70%) can occasionally be found. Such video poker games are offered at a low denomination and may be placed close to the front of the casino, as a loss leader. In some cases, professional players will target video poker games that offer these great odds. It should be clear that if a casino is using a “cash back for points” marketing system, together with offering a video poker game with a break-even house edge, the expert player will turn the house edge well in his favor.

The house edge is an exact number that can be worked out precisely for most games. For many games, the edge can be determined by using a spreadsheet. For some games, the house edge is determined by doing an exhaustive analysis of every possible combination that can occur in the game. This may require extensive spreadsheet analysis. Typically the mathematics for slot machines is done this way. The analysis may require a computer to go through each combination of events, work out the proper strategy for that combination, and keep track of the overall results. This is commonly referred to as “running a cycle.” Video poker is analyzed by running a cycle. 

Whenever possible, the house edge is obtained by a complete spreadsheet analysis or by running a cycle (or both, just to be sure). A computer simulation is often used to confirm the exact theoretical results. For a few games, the toughest games, the rules and strategy are so complex that the house edge can only be approximately determined by computer simulation. Once casino management knows the house edge, they will have a sense for how much money they can make from the game based on the total wagers. But, the house edge tells other stories as well. If the house edge is too close to 0%, then the casino won’t make enough money from the game. If the house edge is too high, then players will lose their money too fast and abandon the game, and once again the casino won’t make enough money from the game.

The house edge is another balancing act, just like the “hit frequency” and “payout” dilemma described in the lottery examples. The house edge has to be low enough to make players want to play the game and high enough so that the casino earns a reasonable amount of profit from the game.

The standard table games consisting of baccarat, blackjack and craps, all offer wagers with a house edge under 1.5%. There are very few table games that make it into a casino with a house edge under 1.5% and those that do are mostly blackjack variants. Well known examples are Spanish 21, Super Fun 21, and Blackjack Switch. For example, with liberal rules and perfect play, Blackjack Switch has a house edge under 0.20%. In practice, due to player errors, Blackjack Switch often returns in excess of 3%. Spanish 21 and Super Fun 21 also have huge error rates. Blackjack variants also move very quickly, generating many more decisions per hour than other games, making the games affordable for the casino.

Roulette maintains its popularity, even though every wager at roulette has a fixed house edge of 5.26%. The house edge can easily be computed by hand as follows. First note that without the 0 and 00 spots on the wheel, the payouts for roulette are designed to be absolutely fair based on a wheel with 36 spots: a 0.00% house edge. By putting both 0 and 00 on the wheel, there are two bad spots on the wheel out of 38 total spots. So, for double-0 roulette, the house edge is 2/38 = 5.26%. For single-0 roulette, the edge is 1/37 = 2.70%.

It is not quite true that every roulette wager has the same house edge; there is an exception that we note. Double 0-roulette sometimes offers a wager called “first 5” that pays 6-to-1. This wins if any of 0, 00, 1, 2, or 3 hits. It loses if any other number is hit. The house edge for this wager is 3/38 = 7.89%. The great majority of roulette players know better than to take this bet, just as most blackjack players know to never take insurance.

For games with side bets, it is usually the case that the main game has a lower house edge than the side bet. Moreover, the lower the edge on the main game, the higher the house edge on the side bet. Side bets do not have the same practical limitations in the size of their payouts or house edge as the main bet. A 3% edge on the main game can easily go with a 7% house edge on the side bet, for example.

As an example, consider the Pair Plus bet on Three Card Poker. The main game in Three Card Poker (with the standard 1-4-5 bonus table) has house edge 3.37%. Shuffle Master includes pay tables for the Pair Plus with house edges varying from 2.32% to 7.28%. When Three Card Poker was first introduced, the 2.32% version of Pair Plus was most common. Now the 7.28% version is the main one used. There is

logic to this increase; from the casino’s side they earn a lot more from a well established and loyal player base; from the player’s side the change is just “cosmetic” and they don’t notice it.

For our lottery examples, it is easy to compute the house edge by running a cycle. That is, we see what happens after every ticket is sold for each lottery. Whether it is lottery 1 or lottery 2, there are 1 million tickets, and each cost $1. So a full cycle corresponds to an investment of $1,000,000 (1 million dollars) from the players. For each lottery, $900,000 is returned in prize money: there are either 9 tickets worth $100,000 each, or 900,000 tickets worth $1 each. For each lottery, the state keeps the remaining $100,000. Thus, for each lottery, the house edge is ($100,000) / ($1,000,000) = 10%.